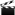35 replies to this topic

[Winslow Strong]

Hi Longecity,

My interest was recently piqued to investigate telomere science as it pertains to health by the offer from TeloMe of cheap DTC telomere length (TL) measurements.

http://www.indiegogo...ong-term-health

I wrote up my analysis of telomere science and TeloMe on my website:

http://biohackyourse...rs-with-telome/

My findings were not definitive, and much uncertainty remains regarding the utility of TeloMe's saliva-based test of average TL (averaged over any and all cells found in your salivary sample). I'd appreciate some feedback on this point, or on anything else relevant to telomere science and TeloMe's service.

I've been in communication with TeloMe, and they support the possibility of their test being a marker for Leukocyte TL with the following references:

http://www.nature.co...l/1702170a.html
http://www.ncbi.nlm....pubmed/22118990
http://www.ncbi.nlm....pubmed/23511462

IMO these all just provide circumstantial evidence of the potential utility of the measurement.

Do you find the test convincing?

Thanks for any feedback,
Winslow

[hav]

That certainly does look interesting. I guess the obvious question is the comparative accuracy of a saliva test compared to a blood test. Which I'd also like to see some informed feedback on. I have the impression that the telomere length of white blood cells is an important immune system health indicator and wonder if a saliva based test would measure enough of that; they don't seem to indicate what the estimated ratio of white blood cells to other cells in their test results. Also, although the $99 cost of the average telomere length analysis seems pretty attractive cost-wise, its probably not as good an indicator as the $499 test which shows the short and long telomere distribution. I wonder if this more expensive analysis reports results by cell types?

I also imagine that until the number of tests they run increase in number in each category, that the age-to-telomere length they report in their database won't have much relevance: right now only 2 people have ordered the $499 complete distribution analysis and only 7 have ordered the $99 average one. But, fwiw (which may be limited), I don't expect it'll take long for numbers to grow for the inexpensive test.

I supplement with supposed telomere length enhancing and protective supplements but have never had easy access to a telomere test. This seems pretty affordable and easy. And I like the fact that they're affiliated with PGP. Which test will PGP be using? Looking forward to some more feedback here.

Howard

Edited by hav, 04 April 2013 - 03:43 PM.

[Winslow Strong]

The last reference I posted suggests it may not matter that much what tissue the telomeres are being measured from, as long as apples are compared to apples in tests across time. Of course the benchmark is leukocyte TL, and it would be preferable to measure that, and preferable also to have the length distribution.

I'm curious what you supplement with? Straight up astralagus? A derivative? or something else altogether?

Id like to see the gene therapeutic approaches that have been successful in turning on telomerase in mice start to be tested in monkeys ASAP. People are dying here! :P

[hav]

Yes, Astragalus extract standardized to 70% polysaccharides. And lately, epithalon too. One member here reported good test reading improvements after switching from as4 and cycloastragenol, if I remember correctly, to a similar astragalus extract. I followed suit on the strength of his report. But I haven't seen anyone post their telomere length test results here after taking epithalon yet. Be helpful if a testing service also recorded what supplements are being taken and reported any correlations. Perhaps PGP might do that.

Howard

[Winslow Strong]

Yeah I think PGP will give us a lot more understanding of factors that correlate with changes in telomere length over time. Too bad that it probably won't be randomized interventions to test. We need to figure out how to start crowdsourcing experiments like that. Would still be problems with compliance tho for the groups.

[Marios Kyriazis]

Yeah I think PGP will give us a lot more understanding of factors that correlate with changes in telomere length over time. Too bad that it probably won't be randomized interventions to test. We need to figure out how to start crowdsourcing experiments like that. Would still be problems with compliance tho for the groups.

From what I have seen the TeloMe testing is the best available for the public (not the ideal approach but the best that currently exists, under the circumstances). I am trying to get together a group of people who are using this measurement (or any other reliable way of telomere testing), in order to conduct a clinical trial of a possible telomere-enhancing lifestyle strategy (NOT a supplement). If you or anyone is interested, please PM me.

[Mind]

Hey Marios, I would be interested in participating in a lifestyle vs. telomere study. As long as the cost was only $100 for the telomere test (or free, if there was a grant or some other type of funding)

[Winslow Strong]

I'm certainly curious about the experiment also. It depends a lot on what type of treatments that you have in mind. For example, I wouldn't stop consuming omega-3s just for the sake of being a control in an experiment to see if omega-3 consumption lengthens telomeres. I wouldn't pay for TA-65 based on current evidence and pricing either.

But there might be interesting treatments that I would be willing to be a control or exp for. Want to elaborate a bit?

[Mind]

I hate to speak for Dr. Kyriazias, but from his comment, I thought he was proposing a lifestyle (sleep, food, exercise) experiment, not anything to do with supplements.

[Winslow Strong]

Whatever the treatment is, the problem could still remain in principle. If there's moderate evidence suggesting that such a practice is generally unhealthy, or bad for telomeres length, then it seems like a very small benefit to advance science in this way while taking a large risk that you are harming yourself.

On the other hand, if theres no reason to believe that the treatments would have an effect on TL, then they aren't really compelling hypotheses to test.

I can't say for sure whether I would or would not try it until he says what the specific treatments would be, but a priori finding treatments that are both compelling to test and that a rational individual who cares about their health wouldn't want to implement anyway seems like a difficult problem to surmount.

Maybe something like this would work: Since meditation has been shown to have some benefit in a few trials, an experiment could be done with one group trying one form of meditation and the other a different one.

[hav]

...I am trying to get together a group of people who are using this measurement (or any other reliable way of telomere testing), in order to conduct a clinical trial of a possible telomere-enhancing lifestyle strategy (NOT a supplement). If you or anyone is interested, please PM me.

Whatever the treatment is, the problem could still remain in principle. If there's moderate evidence suggesting that such a practice is generally unhealthy, or bad for telomeres length, then it seems like a very small benefit to advance science in this way while taking a large risk that you are harming yourself.

On the other hand, if theres no reason to believe that the treatments would have an effect on TL, then they aren't really compelling hypotheses to test.

Got to agree with Mind. Doesn't sound like the study involves treatment. He says it doesn't involve supplements and only involves life style. I assume that might consist of stress relieving lifestyle adjustments and perhaps a natural high-fiber diet.

Howard

[Marios Kyriazis]

...I am trying to get together a group of people who are using this measurement (or any other reliable way of telomere testing), in order to conduct a clinical trial of a possible telomere-enhancing lifestyle strategy (NOT a supplement). If you or anyone is interested, please PM me.

Whatever the treatment is, the problem could still remain in principle. If there's moderate evidence suggesting that such a practice is generally unhealthy, or bad for telomeres length, then it seems like a very small benefit to advance science in this way while taking a large risk that you are harming yourself.

On the other hand, if theres no reason to believe that the treatments would have an effect on TL, then they aren't really compelling hypotheses to test.

Got to agree with Mind. Doesn't sound like the study involves treatment. He says it doesn't involve supplements and only involves life style. I assume that might consist of stress relieving lifestyle adjustments and perhaps a natural high-fiber diet.

Howard

The term 'lifestyle' involves many aspects other than diet, stress, exercise etc. It may be better described as a 'Worldview' rather than the ordinary meaning of the term.

The lifestyle changes I am suggesting refer to the way we behave towards technology, and encourage increased engagement and integration with digital technology. Research under way shows that this may have a direct impact upon cell immortalisation mechanisms, involving, among others, telomerase.

[Winslow Strong]

I'm with you that that could make a big difference. But that doesn't seem like a good thing to try to run a crowd-sourced trial on. We will all be left to our own devices to try to implement such lifestyle changes. Compliance may be poor, and on top of that may be difficult to verify.

[Marios Kyriazis]

I'm with you that that could make a big difference. But that doesn't seem like a good thing to try to run a crowd-sourced trial on. We will all be left to our own devices to try to implement such lifestyle changes. Compliance may be poor, and on top of that may be difficult to verify.

The general idea for the trial was to test the telomeres of a group of people who live in a community with poor digital engagement (such as a remote European agricultural village) and compare this with telomere length of a matched group of people who are embedded within technology (i.e. central London or New York executives). It is relatively easy to match theses groups of people despite the apparent difference in lifestyle. If my hypothesis is correct, the telomeres of the second group will be, on average, longer compared to those of the first group.

In practice, this will be rather complicated, because telomeres from germ cells (i.e. sperm) will need to be examined as well, and ideally also telomeres from neurons. But this is the general idea.

[Winslow Strong]

Oh, I see. This isn't really an experiment then, its a prospective cohort study. Well I for one would certainly be happy to contribute my data from TeloMe to that cause. Shall I get in touch when I receive it?

[Marios Kyriazis]

Yes, please do, together with details about yourself and your lifestyle. We only need a relatively small number of participants at this stage,

[Mind]

Dr. Estep will be a guest on the LongeCity Now podcast, coming up soon.

[Mind]

The Estep interview is now available. A lot of good info!

Perhaps the Telome project could coincide with what has been proposed here. All we would need is for the Telome volunteers to answer a lifestyle questionnaire.

[DorianGrey]

Any news from TeloMe? I've supported the campaign (money was withdrawn) to get at least my average telomer length as a baseline before I try some inducers. Too bad they missed the 15k goal (close call), I would have gone to the more elaborate histogram test for different cell type. Maybe they can freeze a sample so we could check again in lets say in 5 years. But so far I just got one vague email pertaining to the end of the campaign.

Does anyone know the precision and accuracy of the methods TeloMe uses?

@Marios: I don't think the Hayflick limit is an issue for neurons. Germ cells have Telomerase on and lengthen the telomers over time - so it's not bad to have an older father, although mutations may become an issue with really old fathers.

[DorianGrey]

I am expecting the shipment of the test kit from Telome now, got the info this week. I'll start with the basic test and they say you can upgrade to distribution anytime, apparently for the same sample. I also got some info on precision. You won't see year to year differences in the basic test but will have a lot of data when upgrading.

[Winslow Strong]

I was emailing with Dr Estep, and he wouldn't tell me anything about precision. Did you get some numbers? E.g. a standard deviation in base pairs? If so, from where?

[DorianGrey]

He stated "tens of nucleotides" by certain cell types in the upgraded test. What I've seen so far (other labs) was always reported at 0.1kB.

Edited by DorianGrey, 31 May 2013 - 04:22 PM.

[Turnbuckle]

When you look at the plot published by TeloMe of length vs age, it's apparent that the results will be almost meaningless, for after the age of 60, the curve is flat. And even before that, the scatter is large.



Here's a plot of over a thousand data points that shows more dramatically how weakly telomere length is correlated with age--



Imagine a life insurance company trying to base rates on this.

[DorianGrey]

@turnbuckle: This is true for average length. The graph above just states there is a distribution by age group, there are upper and lower limits and these go down with age. The Telome graph has data points past 100 years, the other only to 75 years. Telome uses 0kB and the other 5kB, so visually Telome looks better but shows similar scattered data (noticed they have data point above 10kB, the other one doesn't which could be an accuracy issue of a method).


I am quite sure I will upgrade once the first results show up. I just didn't want to invest 300$ in a start-up that's just coming out with the service. I think you have to look at the shortest telomers because these are known to cause severe structural DNA damage and the inducers seem to act on those, so seeing any difference I need to establish a baseline. My primary objective is health span, life span is an added benefit. In our Western society many people extend their life span with medication but often at the price of a poor quality of living, which is now becoming more and more a parameter in the pharmaceutical industry when new drugs are brought to market.

[niner]

The lack of correlation between average length and age *might* just mean that at any given age, there are people who are healthy and people who aren't. It would be interesting to see what the correlation was between average length and health status. Perhaps more interesting would be to see the correlation between fraction of critically short telomeres and health status. Since telomerase doesn't work well on telomeres that are longer than the critical length, it's the only thing we can change, but fortunately it also seems to be the most important telomere statistic. I'm excited about Telome not just because it's the first easy and affordable test, but even more because it provides the fraction of critically short telomeres at a reasonable price.

[DorianGrey]

niner, that's exactly my view. I just would like to learn exactly which inducer have the best benefit/price ratio and are safe to use (e.g. Nicotine is cheap, but not safe). I got my Telome testing kit yesterday, two (?) tubes with preservatives, a funnel to collect spit and good instructions, along with an envelope and protective material for shipping. I will return it next Monday, I hope it's not going to be extremely hot during shipping to have impact on the results.
Telomer length is probably the best long-term indicator of healthy or unhealthy life-style. I believe it's reflecting diet and inflammation levels, also genetic and epigenetic factors. All of these contribute to longevity and health. The graph of average length is still interesting, supposing a Gaussian bell curve distribution. So the people with low mean most likely also have some extremely short telomers in some cells. It may not always have clinical consequences, but I cannot imagine there is no correlation with health when there are so many senescent cells. On the other hand, you could have a high average but issues with one or two cell types only which doesn't necessarily lower the mean too much.

Edited by DorianGrey, 14 June 2013 - 08:34 PM.

[hav]

niner, that's exactly my view. I just would like to learn exactly which inducer have the best benefit/price ratio and are safe to use (e.g. Nicotine is cheap, but not safe). I got my Telome testing kit yesterday, two (?) tubes with preservatives, a funnel to collect spit and good instructions, along with an envelope and protective material for shipping. I will return it next Monday, I hope it's not going to be extremely hot during shipping to have impact on the results.
Telomer length is probably the best long-term indicator of healthy or unhealthy life-style. I believe it's reflecting diet and inflammation levels, also genetic and epigenetic factors. All of these contribute to longevity and health. The graph of average length is still interesting, supposing a Gaussian bell curve distribution. So the people with low mean most likely also have some extremely short telomers in some cells. It may not always have clinical consequences, but I cannot imagine there is no correlation with health when there are so many senescent cells. On the other hand, you could have a high average but issues with one or two cell types only which doesn't necessarily lower the mean too much.

Hi, Dorian. The uncertainty on actual shipping/processing dates and possible weather and temperature conditions was the main reason I passed on the earlier promotion. Now that I see ambient temperatures have been pretty low I regret not having jumped on it. But with my luck, if I ordered it now, I'd probably get the shipping kit in July with outside temperatures in the 90's. Hoping they run another special with guaranteed shipping in the Fall or Winter.

Howard

[BobSeitz]

Recently, when I visited the Repeat Diagnostics website, I was struck by the telomere length curves they showed for a couple of their clients. These curves exhibit two notable features.

(1) There's a standard telomere length curve shape that describes how, on average, everyone's telomeres shorten as a function of time. We lose telomere base pairs the fastest at birth (at a rate of 140 base pairs a year?), with the rate steadily slowing as we work our way through childhood and early adulthood. Somewhere around 55 or 60, there's an inflection point in the curve where the curvature shifts from positive to negative. Our rate of telomere erosion in at its lowest point at that age, with a rate of telomere loss of about 35-36 base pairs a year. (The curve is nearly flat from 40 to 70, with telomere loss rate <40 base pairs per year.) After the age of 70, the curve begins to fall ever faster.

(2) There are wide variations in the initial lengths of people's chormosomes at birth. This dispersion of individual telomere lengths at birth looks as though it might be Gaussian, with a standard deviation of, maybe, 1,000 base pairs. If so, the 90th and 10th percentiles are about ±1.27 standard deviations from the mean, and the 99th and 1st percentiles would be about ±2.06 s. This means that about 1 in 10 of us are born with telomeres with a median telomere length about 11,700 base pairs long, and 1 in 100 of us are bequeathed telomeres with a median extension of about 12,500 base pairs. One in 700 of us would greet the world with about 13,400 base pairs. Averaged over populations these telomere length advantages remain unchanged throughout life!

This may help to explain the scatter on telomere measurement charts. We're, maybe, not looking at fluctuations in telomere lengths over time, but at individual differences in birth date telomere lengths that are conserved throughout life.

A third-degree polynomial (a cubic) is the simplest polynomial fit that can describe this curve. I've fitted a cubic,
Telomere Length (TL) = d - at³ - bt² - cy,
to this curve as best I could by visually guesstimating values from the chart. The coefficients for this polynomial fit for the middle (average) curve are:
a = 9.5833333 = 9 7/12ths
b = -17.25
c = 14.0666667 = 14 1/16th
d = 10.4 kilobase pairs

d is the initial value for the middle (third) curve, and it appears to be somewhere in the neighborhood of 10,400 base pairs for the average person at birth.

To keep these numbers moderate, I measured time as a fraction from 0 to 1, where 1 = 100 years, and I measured telomere lengths in kilobase pairs.

Since the other four curves have identical shapes, they may be modeled by adding or subtracting 1,270 base pairs to "d" to get to the 90th or 10th percentiles, and by adding or subtracting 2,060 base pairs to "d" to accommodate the 99th and 1st percentiles respectively. (a, b, and c would be the same for all five curves.)

The characteristics of this cubic fit curve are that it's anti-symmetric around the inflection, which I took to be 60 for this cubic fit. Differentiating the cubic to get the slope (annual rate of telomere loss) yields:
Rate of telomere change = - 3 at² - 2 bx - c.

At t = 0,
Rate of telomere change = -c. But c = 14,067 base pairs per century, or 140.67 base pairs per year. So the rate of telomere change at birth for everyone is about:
Rate of telomere change = -140.67 base pairs a years
Because this is a cubic fit and is anti-symmetric about age 60 (0.6 centuries), the slope at age 120 is equal to the slope at birth: -140.67 base pairs a years. This is an artifact (limitation) of the model.

I'm working a 4th-degree polynomial fit (a quartic) but I haven't finished it yet.

A logical next step would be to contact Repeat Diagnostics to seek more accurate numbers for this curve fit (although high-fidelity numbers may be contingent upon additional data-gathering over larger populations).
It's tempting to relate telomere lengths at birth to life spans, but I think it must be more complicated than that. If you smoke heavily and work in an asbestos factory, I suspect that you may get lung cancer even if you were blessed with long telomeres at birth. Also, identical twin studies suggest ot me a meaningful role for lifestyle choices.

[DorianGrey]

Before doing too much modelling on average telomer length, take a look at these publications:
http://www.agemed.or...US/Default.aspx
http://www.pnas.org/...00/F3.large.jpg

Edited by DorianGrey, 24 June 2013 - 02:47 AM.

[blood]

I got my Telome testing kit yesterday, two (?) tubes with preservatives, a funnel to collect spit and good instructions, along with an envelope and protective material for shipping. I will return it next Monday

Did you get your results back?

Edited by blood, 09 August 2013 - 10:33 PM.